Cylindrical projections

Conceptually, cylindrical projections are created by wrapping a cylinder around a globe and projecting light through the globe onto the cylinder.

Cylindrical projections represent meridians as straight, evenly-spaced, vertical lines and parallels as straight horizontal lines. Meridians and parallels intersect at right angles, as they do on the globe.

Depending on the placement of the light source, the parallel spacing is different.

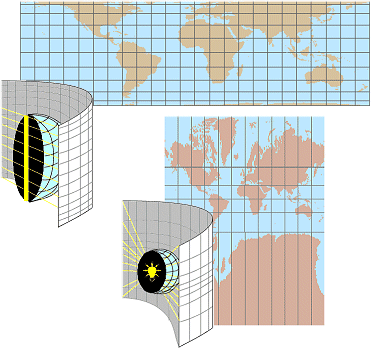
Top: The light rays are perpendicular to the earth's polar axis. Parallel spacing decreases toward the poles. Bottom: The light rays emanate from the center of the globe. Parallel spacing increases toward the poles.

Here's a more detailed look at how a Central Cylindrical projection (like the bottom example in the previous graphic) renders the graticule.

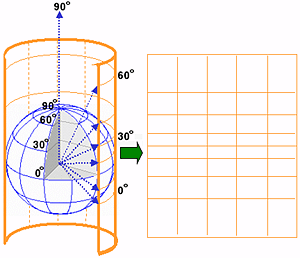
The poles on a Central Cylindrical projection can't be displayed because the projected 90° latitude never contacts the cylinder. In fact, this projection is never used because of its extreme distortion. Projections that resemble it, such as the Mercator, are used, however.

Different cylindrical projections have been developed to preserve different spatial properties. Some preserve area, some shape, and some true distance along their meridians.

The most famous of all map projections—the Mercator—is a cylindrical projection. Like the Central Cylindrical, the Mercator is also unable to project the poles and creates severe area distortion at latitudes near the poles. Its virtues are that it is conformal and that any straight line drawn on it is a true compass bearing. This property has made the Mercator (and still makes it) an invaluable nautical chart.